奇异位形严重影响Gough-Stewart并联机构的运动及力传递性能,机构若处于奇异状态,将严重失稳并导致其失控甚至被损坏. 因此,Gough-Stewart并联机构应位于远离奇异位形的区域工作 ^{[ 1]} .

Li等 ^{[ 2]} 指出,有必要得到并联机构的奇异轨迹表达式及其图形化描述,从而为进一步研究如何避开奇异位形提供必要的依据. 众所周知,Gough-Stewart并联机构具有6个自由度,机构的奇异轨迹曲面是一个嵌入六维空间的超曲面,故很难将其实施图形化描述. Li等 ^{[ 2]} 以 ZYX-欧拉角为姿态参数,探讨了Gough-Stewart并联机构在若干三维子空间内的奇异轨迹曲面. 基于 ZYX-欧拉角为姿态参数,Huang等 ^{[ 3]} 得到了该并联机构位于给定姿态时的位置奇异轨迹曲面. Li等 ^{[ 4]} 研究了该类型并联机构处于给定姿态时,位于三维位置空间内的奇异轨迹分布及其特征平面上的奇异轨迹的性质. Pendar等 ^{[ 5]} 提出利用平面几何中的Ceva定理研究了三角平台型Gough-Stewart并联机构的奇异位形. Bandyopadhyay等 ^{[ 6]} 基于欧拉参数为姿态参数,得出6-6型Gough-Stewart并联机构位于R ³空间上的奇异轨迹,并将欧拉参数转化为球面描述形式,得到了该机构位于给定位置时的姿态奇异轨迹6次多项式. Jiang和Gosselin ^{[ 7- 8]} 以 ZYX-为姿态参数,给出了3-3 型Gough-Stewart并联机构的非奇异姿态空间的搜索算法. Cheng等 ^{[ 9]} 以单位四元数为姿态参数,分别给出了Gough-Stewart并联机构位于给定姿态时的位置奇异轨迹和位于给定位置时的姿态奇异轨迹的图形化描述.

文献[ 10- 12]提出利用运动规划的方法避开机构的奇异位形. 王玉新等 ^{[ 13]} 通过研究并联机构构型分岔特性,提出了一种利用扰动函数来规避并联机构转向点奇异的方法. 文献[14-17]提出无奇异工作空间的求解方法,机构在无奇异工作空间内工作将不会出现奇异位形. 无奇异工作空间实际上也是机构的有效工作空间,它的大小在某种程度上也决定了机构性能的好坏. 如何高效、准确地得到并联机构的有效工作空间,同时揭示机构构型参数、位姿参数等对机构有效工作空间大小的影响情况,是各类并联机器人机构结构与构型参数设计时的一项重要课题. 文献[18-19]给出了动、定平台为相似六角平台并相反布置的Gough-Stewart并联机构的姿态能力以及姿态空间算法,但对具有更加一般结构形式的动、定平台为非相似六角平台的姿态能力及其影响因素未做进一步的研究,并且上述文献给出的一般离散算法求解效率较低.

鉴于非相似型Gough-Stewart并联机构在结构型式上更具有一般性,在工程应用领域中也具有更广泛的应用前景,本文将进一步研究该类型并联机构的姿态奇异与姿态能力. 以单位四元数描述机构动平台的姿态,得到机构位于给定位置时的具有一般符号形式的姿态奇异轨迹方程,考虑到姿态奇异轨迹分布特性,给出一种计算机构姿态能力的高效求解算法,并进一步研究机构构型参数和位置参数对机构姿态能力的影响情况. 姿态能力可以衡量机构位于给定位置时非奇异姿态空间的大小,也可作为优化机构几何构型参数时的一项性能指标.

1 三维姿态奇异轨迹描述

六自由度Gough-Stewart并联机构的结构简图如 图1所示,其动定平台为2个非相似型的半对称正六边形 B ₁ B ₂… B ₆, C ₁ C ₂… C ₆ ( i=1,2,…,6),并通过六根相同的球副 -移动副 -球副(或万向铰)支链( B _iC _i )相连 . B _i 和 C _i 分别为动定平台的6个顶点, A _j ( j=1,3,5)为定平台六边形长边的交点 .

图  1  Gough-Stewart并联机构的结构简图

Figure  1.  Schematic of the Gough-Stewart parallel mechanism

下载: 全尺寸图片 幻灯片

图1中: P为机构动平台几何中心点; O为机构定平台的几何中心点; β _m为动平台上边 B ₄ B ₅对应的中心角,0°≤ β _m≤120°; β _b为定平台上边 C ₁ C ₂对应的中心角,0°≤ β _b≤120°; R _m为动平台外接圆半径; R _b为定平台外接圆半径.

为分析并得到机构的奇异轨迹方程,在机构动平台上建立固连坐标系 P-xyz,在定平台上建立固定参考系 O-xyz. 将动平台中心点 P作为动平台运动的位置参考点,设点 P在固定参考系 O-xyz中的位置矢量记为 P=[ X, Y, Z] ^T;记动平台各铰点 B _i 在动坐标系 P-xyz中的位置矢量为 b _i ( i=1, 2, …, 6),记 B _i 在固定坐标系 O-xyz中的位置矢量为 B _i ( i=1, 2, …, 6);定平台各个铰点 C _i 在固定参考系 O-xyz中的位置矢量记为 C _i ( i=1, 2, …, 6) .

定义机构的初始姿态,其满足条件: 1) 给定机构动平台参考点 P的位置; 2) 动定坐标系的相应坐标轴相互平行.

机构的几何构型取决于4个参数: R _m、 R _b、 β _m、 β _b. 机构动定平台的形状分别取决于 β _m、 β _b,如 表1所示.

表  1  动定平台的形状与中心角大小的关系

Table  1.  Relationship between the shapes of the two platforms and the central angles

中心角大小	对应边长度	平台形状
β m,b=0°	0	等边三角形
β m,b<60°	较短边	半对称正六边形
β m,b=60°	个边长均相等	正六边形
β m,b>60°	较长边	半对称正六边形
β m,b=120°	最大值	等边三角形

下载: 导出CSV 

| 显示表格

在此,以具有3个独立参数的单位四元数 Q=q ₀ +q ₁ i+q ₂ j+q ₃ k, $\begin{array}{c}{q}_0^2\end{array}$ + $\begin{array}{c}{q}_1^2\end{array}$ + $\begin{array}{c}{q}_2^2\end{array}$ + $\begin{array}{c}{q}_3^2\end{array}$ =1描述该机构动平台的姿态 . 其表示机构动平台以初始姿态绕通过 P点且相对于固定坐标系矢量方向 q ₁ i+q ₂ j+q ₃ k的轴旋转角度 ϕ=2arccos q ₀所形成的姿态 . 此外,规定 q ₀≥0,这样该单位四元数与刚体的姿态为一一对应关系,且避免了以欧拉角等描述姿态时所引起的以旋转矩阵求解欧拉角逆问题时无全局光滑解的问题 ^{[ 20]} . 基于单位四元数的旋转矩阵为

R= $\begin{array}{c}\left(\begin{array}{ccc}{q}_0^2+q_1^2-q_2^2-q_3^2& 2(q_1{q}_2-q_0{q}_3)& 2(q_1{q}_3+q_0{q}_2)\\ 2(q_1{q}_2+q_0{q}_3)& q_0^2+q_2^2-q_1^2-q_3^2& 2(q_2{q}_3-q_0{q}_1)\\ 2(q_1{q}_3-q_0{q}_2)& 2(q_2{q}_3+q_0{q}_1)& q_0^2+q_3^2-q_1^2-q_2^2\end{array}\right)\end{array}$ (1)

由坐标变换原理不难得到 B _i 与 b _i 满足

B _i=Rb _i+P (2)

将式(1)带入机构奇异位形判别矩阵

J ^T = $\begin{array}{c}\left(\begin{array}{cccccc}\frac{B_1-C_1}{|B_1-C_1|}& \frac{B_2-C_2}{|B_2-C_2|}& \frac{B_3-C_3}{|B_3-C_3|}& \frac{B_4-C_4}{|B_4-C_4|}& \frac{B_5-C_5}{|B_5-C_5|}& \frac{B_6-C_6}{|B_6-C_6|}\\ \frac{C_1\times B_1}{|B_1-C_1|}& \frac{C_2\times B_2}{|B_2-C_2|}& \frac{C_3\times B_3}{|B_3-C_3|}& \frac{C_4\times B_4}{|B_4-C_4|}& \frac{C_5\times B_5}{|B_5-C_5|}& \frac{C_6\times B_6}{|B_6-C_6|}\end{array}\right)\end{array}$ (3)

若令式(3)所示矩阵的行列式等于零,即可得到机构奇异位形关于位姿参数的解析表达式

F( A, B) =0

式中: A表示机构动平台的位置参数; B表示机构动平台的姿态参数 .

将式(1)(2)带入式(3),并注意到 q ₀ = $\begin{array}{c}\sqrt{1-q_1^2-q_2^2-q_3^2}\end{array}$ ,展开并化简矩阵 J ^T的行列式,并令其等于0,得到该机构位置参数 P给定时的姿态奇异轨迹一般符号解析表达式

f ₁ $\begin{array}{c}{q}_1^6\end{array}$ +f ₂ $\begin{array}{c}{q}_1^5\end{array}$ q ₂ +f ₃ $\begin{array}{c}{q}_1^5\end{array}$ q ₃ +f ₄ $\begin{array}{c}{q}_1^5\end{array}\begin{array}{c}\sqrt{1-q_1^2-q_{21}^2-q_3^2}\end{array}$ +… +f ₆₉ $\begin{array}{c}{q}_2^2\end{array}$ +f ₇₀ q ₂ q ₃ +f ₇₁ $\begin{array}{c}\sqrt{1-q_1^2-q_{21}^2-q_3^2}\end{array}$ +f ₇₂ $\begin{array}{c}{q}_3^2\end{array}$ +f ₇₃ =0(4)

式中: f _i ( i=1, 2, …, 73)均是机构构型参数 R _m、 R _b、 β _m、 β _b以及位置参数( X, Y, Z)的显示表示 .

进一步观察发现,式中多项含有 $\begin{array}{c}\sqrt{1-q_1^2-q_2^2-q_3^2}\end{array}$ ,姿态参数 q ₁、 q ₂最高达6次, q ₃的最高次达4次.

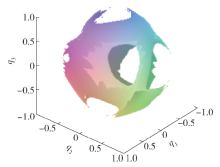
当给定机构的构型参数 R _m=1、 R _b=2、 β _m=75°、 β _b=105°,机构位于给定位置(0, 0, 4)时关于姿态参数( q ₁, q ₂, q ₃)的姿态奇异轨迹在笛卡儿坐标系中的三维图形化描述如 图2所示.

图  2  机构位于给定位置的姿态奇异轨迹

Figure  2.  Orientation-singularity locus for a given position

下载: 全尺寸图片 幻灯片

2 姿态奇异性质分析

为便于阐述机构处于给定位置时姿态奇异位形的性质,在此给出“姿态原点”的概念:当机构位于“姿态原点”时,应满足机构动、定坐标系具有相同的姿态,机构动平台的姿态参数为(0, 0, 0) .

当机构处于姿态原点时,将机构的位置参数 A=( X, Y, Z)及姿态参数 B=(0, 0, 0)带入式(4)便可得到机构位于初始位形时的奇异轨迹表达式

gZ ³ =0(5)

式中: g由机构构型参数 R _b、 R _m、 β _b、 β _m决定,并且只要 β _b+ β _m≠120°,即只要机构不满足结构奇异时,必有 g≠0 .

式(5)不含位置参数 X、 Y,若使式(5)成立,必然有 Z=0 . 也即是说,当 Z≠0也即动、 定平台不重合且机构位于姿态原点(0, 0, 0)时,无论位置参数 X、 Y为何值,机构的雅可比矩阵行列式必不等于0,即机构不会处于奇异位形 . 另一方面,机构处于给定位置时的姿态奇异轨迹方程(4)必然关于姿态参数( q ₁, q ₂, q ₃)连续,因此,当给定机构位置参数( X, Y, Z),机构在关于姿态参数 B的姿态原点(0, 0, 0)附近必然存在一个无奇异姿态空间. 从 图2可以看出,机构在若干给定位置处的姿态原点附近,确实存在一个无奇异姿态空间. 综上所述,可得出以下重要定论:

定论 对于非结构奇异性六自由度Gough-Stewart并联机构,若动、 定平台不在同一平面内,则机构在以单位四元数表征的三维姿态参数空间原点即(1, 0, 0, 0)附近,理论上必然存在一个无奇异姿态空间.

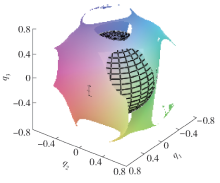
从 图2可看出,由于姿态奇异轨迹分布的复杂性,很难确定以上所述的理论无奇异姿态空间的形状及大小,而并联机器人机构在实际应用时,人们常希望得到具有一定规则形状及大小的姿态空间. 因此,为便于确定理论无奇异姿态空间,在此,将具有规则形状的姿态奇异三维曲面最小内切球作为机构的理论无奇异姿态空间,并且球心为姿态原点( q ₁, q ₂, q ₃)=(0, 0, 0),称此球为无奇异姿态球,如 图3所示. 并将该球的体积大小作为衡量机构处于给定位置时的无奇异姿态变换能力,简称为姿态能力(orientation- capability). 由于球的体积决定于其半径大小,因此,也可将无奇异姿态球半径作为衡量机构姿态能力的大小,无奇异姿态球半径越大,机构的姿态能力越大,理论无奇异姿态空间体积将越大.

图  3  机构位于给定位置的无奇异姿态球

Figure  3.  Orientation-singularity-free ball of the mechanism for a given position

下载: 全尺寸图片 幻灯片

3 姿态能力的数值算法

为便于计算机构姿态能力大小,并考虑单位四元数的性质,将单位四元数转换成下列球坐标表示形式:

q ₁ =r sin α cos βq ₂ =r sin α sin βq ₃ =r cos αα[0, π], β∈[0, 2π], r∈[0,1] (6)

将单位四元数转换为球坐标形式计算的另一个原因是为便于计算姿态能力大小

r= $\begin{array}{c}\sqrt{q_1^2+q_2^2+q_3^2}\end{array}$

将式(6)带入式(4),可得到关于 r、 α、 β的方程

F( r, α, β) =0(7)

因而,计算机构在给定位置时的姿态能力便转化成对于所有的 α、 β取值,满足式(7)时搜寻 r的最小值 r _min问题. Pernkopf等 ^{[ 14]} 综合利用二分法和Sturm原理在Maple软件中来求解无奇异姿态空间. 本文给出另一种计算姿态能力大小的一种通用算法,该算法综合利用逐步搜索算法和二分法来精确地计算姿态能力,算法如下:

步骤1 将机构构型参数 R _b、 R _m、 α _b、 β _m,机构动平台位置参数( X, Y, Z),以及式(6)代入式(4),将式(4)转换成关于 r、 α、 β的式(7).

步骤2 将 α、 β取值范围划分成步长分别为Δ α、 Δ β的一系列微分子空间

α _{i+ 1} =β _i+ Δ α ( i, i+1 =1, 2, …, π/Δ α)

β _{j+ 1} =β _j+ Δ β ( j, j+1 =1, 2, …, 2π/Δ β)

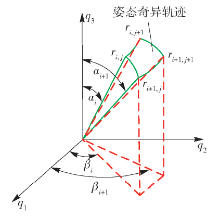
式中: α ₁ =0; β ₁ =0; r ₁ =0 . 在 α=α _i 并 β=β _j 的微分子空间内,式(7)关于 r在[0, 1]的实数解可能不止一个,将其中较小的一个解记为 r _{i , j} ,如 图4所示.

图  4  α=α _i , β=β _j 时的 r _{i , j}

Figure  4.  Notation of r _{i , j} when α=α _i and β=β _j

下载: 全尺寸图片 幻灯片

步骤3 设 α=α _i ( i, i+1 =1, 2, …, π/Δ α),并执行步骤3(a)~(e).

(a) 设 β=β _j ( j, j+1 =1, 2, …, 2π/Δ β),将 r以步长为Δ r从0开始逐步增加,并将第 k个值记为 r ^k ( k=1, 2, …, 1 /( r)) . 将 r ^k 、 α _i 及 β _j 带入式(7)左边,并将其值记为 F( r ^k ) . 若满足 F( r ^k ) ×F( r ^{k+ 1})≤0或 r ^k =1,即可停止步骤3(a),并继续步骤3(b)~3(f).

(b) 若 F( r ^k ) ×F( r ^{k+ 1}) =0,则记 r _{i , j}=r ^{k+ 1}.

(c) 若 F( r ^k ) ×F( r ^{k+ 1}) <0,则必有 r _{i , j} ∈( r ^k , r ^{k+ 1}) . 此时,搜寻 r _{i , j} 即是搜寻如式(7)所示关于未知数 r的在( r ^k , r ^{k+ 1})的解,这样,可利用二分法在搜索区间 [ r ^k , r ^{k +1}]上求解方程的根. 关于二分法的具体算法,在此不再赘述.

(d) 若对于所有的 r ^k 均有 F( r ^k ) ×F( r ^{k+ 1}) >0,则记 r _{i , j}= 1 . 此种情况下,也即 α=α _i 并 β=β _j 时,关于 r的式(7)无解.

(e) 对于其他所有 β值,重复3(a)~3(d).

(f) 对于其他所有 α值,重复3(a)~3(e).

步骤4 将搜索得到的所有 r _{i , j} 取最小值( i=1, 2, …, π/Δ α; j=1, 2, …, 2π/Δ β)便可得到无奇异姿态球半径即姿态能力 r,即有 r=min( r _{i , j} ).

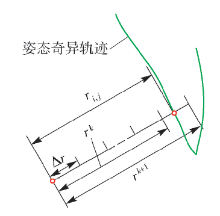
步骤3(c)利用了连续函数的介值定理. 当 α、 β均给定时,式(7)左边是关于 r的连续函数,若 r从 r ^k 连续变化至 r ^{k+ 1},则式(7)左边 F( r)必随着 r的连续变化而变化 . 根据连续函数介值定理,若 F( r ^k ) F( r ^{k+ 1}) <0,则方程 F( r) =0在区间( r ^k , r ^{k+ 1})上至少有一个解,此时,搜寻 r _{i , j} 即是要在( r ^k , r ^{k+ 1})上寻找方程 F( r)=0的根,如 图5所示.

图  5  α=β _i , β=β _j 时正确的 r _{i , j}

Figure  5.  Correct value of r _{i , j} when α=β _i and β=β _j

下载: 全尺寸图片 幻灯片

4 姿态能力大小的影响因素研究

影响机构姿态能力大小的因素主要有动、 定平相对尺寸大小,动、 定平台的几何中心角 β _m、 β _b,以及动平台所处位置( X, Y, Z). 为便于分析,将机构定平台外接圆半径设为 R _b=2,不考虑机构关节运动范围情况下动平台可以到达三维空间内任意位置. 不失一般性,此处机构的长度单位及位置参数单位均为量纲一尺寸.

4.1 动、 定平台几何中心角的影响

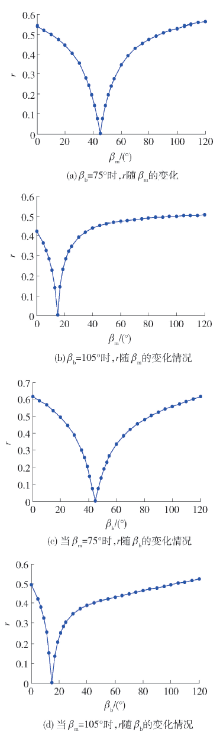
为便于分析机构动、 定平台几何中心角 β _m、 β _b对姿态能力的影响,设动、 定平台外接圆半径比 μ以及动平台位置参数( X, Y, Z)均给定 . 当 R _b=2、 μ=R _m/ R _b=0.5,动平台中心角 β _m和定平台中心角 β _b对机构姿态能力 r的影响情况如 图6所示.

图  6  给定位置(0, 0, 4)时 r随 β _b、 β _m的变化

Figure  6.  r versus β _m for constant β _b at given position (0, 0, 4)

下载: 全尺寸图片 幻灯片

从 图6可以看出,当动、 定平台其中一个中心角给定时,另一个平台越接近三角形形状,则机构姿态能力越大. 但是,众所周知,三角形平台由于制造难度较大,在生产实际中鲜有应用.

此外,当 β _m+ β _b=120°即机构动、定平台为2个相似六边形且对应定点以支链相连时,机构为结构奇异性构型,此时,无论机构处于何种位形,机构均奇异,因此,应避免设计此种结构型式的机构.

4.2 动、定平台相对尺寸大小的影响

假定机构动、定平台中心角 β _m、 β _b以及机构动平台位置参数( X, Y, Z)均给定,动、定平台外接圆半径比 μ=R _m/ R _b便决定了动、定平台相对尺寸大小,其对机构姿态能力影响情况如 图7所示.

图  7  位置(0, 0, 4), β _m、 β _b给定时 r随 μ变化情况

Figure  7.  r versus μ for constant β _m and constant β _b at position (0, 0, 4)

下载: 全尺寸图片 幻灯片

从 图7可以得到以下结论:

1) 其他构型参数给定,当 μ=1时,机构姿态能力接近最大值. 也即是说,当动、定平台具有相同大小的外接圆半径时,机构具有最大无奇异姿态球.

2) 当 β _m/ β _b=0°或 β _m/ β _b=120°时,机构姿态能力随 μ变化而变化的范围很小. 也即是说,当机构动平台或定平台为三角平台时,机构无奇异姿态球大小基本不随动、定平台外接圆半径比变化而变化.

4.3 动平台位置的影响

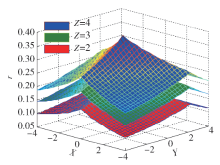
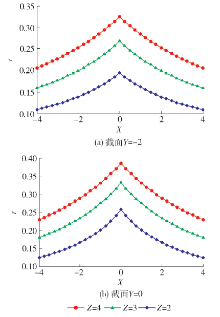
图8描述了机构构型参数均给定时,机构姿态能力 r大小随动平台位置参数不同而变化的情况. 其中 R _m=1、 R _b=2, β _b=90°、 β _m=45°,且Δ X、Δ Y均为0.25,姿态能力变化曲面对应的位置参数分别为 Z=2、3、4 . 图9、10给出了在 Y及 X截面上姿态能力随位置参数变化情况.

图  8  β _b=90°、 β _m=45°时 r随位置参数变化

Figure  8.  r versus position parameters for β _b=90° and β _m=45°

下载: 全尺寸图片 幻灯片

图  9  Y-截面上 r随位置参数 X变化

Figure  9.  r versus position parameter X in Y-sections

下载: 全尺寸图片 幻灯片

图  10  X-截面上 r随位置参数 Y变化

Figure  10.  r versus position parameters X in X-sections

下载: 全尺寸图片 幻灯片

从 图10~12可以得出:

1) 当位置参数 Z给定时,姿态能力随位置( X, Y)变化的曲面关于 X轴对称.

2) 当位置参数 Z给定时,动平台中心点 P越接近 Z轴,机构姿态能力越大,位于 Z轴时,姿态能力达到最大.

图11描述了当( X, Y)给定时,姿态能力随位置参数 Z 变化情况 . 从 图11姿态能力 r随位置参数 Z的变化趋势可以看出,当( X, Y)给定时, Z越大,也即机构动平台距离定平台越远,机构姿态能力越大,但随着 Z的逐渐增大,姿态能力增大的趋势越平缓;当机构动平台位置位于定平台所在平面时,机构无姿态能力.

图  11  ( X, Y)给定时 r随位置参数 Z变化

Figure  11.  r versus position parameters for given ( X, Y)

下载: 全尺寸图片 幻灯片

文献[14]以单位四元数为姿态参数,给出了Gough-Stewart并联机构的无奇异姿态空间的一种数值离散求解算法,研究表明在没有考虑机构结构参数约束条件下,该机构的无奇异姿态空间体积大小随着 Z坐标值的增大逐渐增大. 文献[15]进一步考虑了机构的结构参数约束,给出机构的无奇异姿态空间求解算法,并描述了机构在 Z截面上在若干不同( X, Y)坐标参数下的无奇异姿态空间 . 文献[15]研究也表明机构无奇异姿态空间大小确实关于 X坐标轴对称.

5 结论

1) 论文提出的综合利用逐步搜索和二分法可有效地计算六自由度Gough-Stewart机构无奇异姿态球及姿态能力的大小.

2) 对于六自由度Gough-Stewart机构,其动、定平台为2个相似六边形且对应点相连的结构为结构奇异性构型,应避免设计此种构型参数.

3) 当动、定平台其中一个几何形状给定,另一个平台的几何形状越接近三角形形状,机构的姿态能力越大,即理论上的无奇异姿态球体积越大.

4) 当其他构型参数给定,动、定平台的相对尺寸大小越接近,机构便具有较大的姿态能力. 因此,为使机构具有较大的姿态能力,动、定平台应具有较为接近的相对尺寸.

5) 机构动平台几何中心点距离定平台距离越远且越接近坐标轴 Z时,理论上机构具有的姿态能力越大. 因此,为提高机构在实际工作工程中的姿态能力,在强度及刚度等条件允许情况下,可适当增加各分支杆长度,使得动平台能够在远离定平台的区域工作,从而机构具有较大体积的理论无奇异姿态空间.

6) 作者下一步研究将在基于姿态奇异研究基础上,集中于机构的无奇异姿态运动规划和控制算法等的研究.